RMO 2011 SOLUTIONS

1. Let ABC be a triangle. Let D, E, F be points on the segments BC, CA and AB such that AD, BE and CA concur at K. Suppose \(\frac{BD}{DC} = \frac{BF}{FA}\) and ∠ADB = ∠AFC. Prove that ∠ABE = ∠CAD.

Solution:

Diagram

Given: ABC be any triangle. AD, BE and CF are drawn from A, B, C to BC, CA and AB respectively such that they concur at K and \(\frac{BD}{DC} = \frac{BF}{FA}\) and ∠ADB = ∠AFC.

R.T.P.:  ∠ABE = ∠CAD;

Construction: FD is joined.

Proof: 

Since ∠ADB = ∠AFC; 
hence 180° - ∠BFC = ∠ADB;
=> ∠BFC + ∠ADB = 180°;
=> BDKF is a cyclic quadrilateral (since sum of an opposite pair of angles 180°)

Hence  ∠FBK = ∠FDK (angles in the segment FK in the cyclic quadrilateral BDKF) .... (i)

Since  \(\frac{BD}{DC} = \frac{BF}{FA}\)
=> DF||AC
=> ∠FDA = ∠CAD (alternate angles)
Since  by (i) ∠FBK = ∠FDK
=> ∠ABE = ∠FBK = ∠FDK = ∠FDA = ∠CAD;
=>  ∠ABE = ∠CAD;

Q.E.D.

2.  Let \(a_1 , a_2 , ... , a_{2011}\) be a permutation of the numbers 1, 2, ..., 2011. Show that there exists two numbers j and k such that \(1\le j < k \le 2011\) and |\(a_j - j\)| = |\(a_k -k\)|

Solution:  
Let |\(a_j - j \)| = \(t_j\)

If all the  \(t_j\)-s are different then they will take up each value from 0 to 2010 (included) exactly once.


squaring both sides and adding all terms we get

\(a_1^2 + a_2^2 + ... + a_ {2011}^2 + 1^2 + 2^2 + ... 2011^2  = 2 a_1  1 + 2  a_2 2 + ... + 2  a_{2011} 2011 + (0^2 + 1^2 + ... + 2010^2)\)

The left hand side of the equality is even and the right side is odd. Hence all the numbers from 0 to 2010 cannot appear as values of the 2011 \(t_j\)-s. Hence there must be repetitions.

Proved.

3. A natural number n is chosen strictly between two consecutive perfect squares. The smaller of these two squares is obtained by subtracting k from n and the larger one is obtained by adding ℓ to n. Prove that n - kℓ is a perfect square. 

Solution:

The the two consecutive squares be \(m^2\) and \((m+1)^2\). 
=> n - k = \(m^2\)  ... (i)
     n + ℓ = \((m+1)^2\) ... (ii)
Adding (i) and (ii) we have  (n+ ℓ) + (n-k) = \(m^2 + m^2 + 2m + 1\)
=> 2n + ℓ - k = \(2m^2 + 2m + 1\)
=> ℓ - k = \(2m^2 + 2m + 1 - 2n\)  --- (iii)
Now
(n-k)(n+ℓ) = \(m^2 \times (m+1)^2\)
Hence \((m^2 + m)^2\)
= \(n^2\) + nℓ - nk - ℓk
= \(n^2\) + n(ℓ-1+1) - nk - ℓk
= \(n^2\) + n(ℓ-1) - nk + n - ℓk
= n(n + ℓ - 1 - k) + n - ℓk 
= n(n + ℓ - k - 1) + n - ℓk 
= n(n + 2\(m^2\) + 2m + 1 - 2n - 1) + n - ℓk  .. (replacing ℓ - k by \(2m^2 + 2m + 1 - 2n\) using --- (iii) )
Thus
n - ℓk
= \((m^2 + m)^2  - n( 2m^2 + 2m - n)\)
= \((m^2 + m)^2  - 2n(m^2 + m) + n^2)\)

=> n - ℓk = \((m^2 + m - n)^2\)

HENCE PROVED that  n - ℓk is a perfect square.

4. K is a 20-sided convex polygon with vertices \(A_1 , A_2 , ... , A_20 \)in that order. Find the number of ways in which three sides of K can be chosen so that every pair among them has at least two sides of K between them. For example \(A_1 A_2\) , \(A_4 A_5\) and \(A_11 A_12\) is a permissible triplet, but  \(A_1 A_2\) , \(A_4 A_5\) and \(A_19 A_20\)  is not.

Solution:

Let us divide the set of 3-sides (such that every pair of them has at least two sides between them) into mutually exclusive and exhaustive subsets. Then we will count the subsets effecting the counting of the entire set.

1. Exactly two sides between one pair of selected sides

The two sides along with the two in-between sides create chain of 4 sides. There are 20 such 4-side-chains: \(s_1 s_2 s_3 s_4\) through \(s_20 s_1 s_2 s_3\) where \(s_i\) denotes a side. Note that in each of those 4-side-chains we have selected the first one and the last one. The two in the middle denote the 'gap'.

The third member of the triplet is yet to be selected. There can be two situations:

a) The third side is at exactly 2-side away from one of the two sides selected. Then we basically have a 7-side-chain in which the first, fourth and the seventh are member of the triplet and rest are acting as gap-sides. There are 20 7-side-chains leading to 20 triplets.

b) Third side is strictly MORE than 2-side away from both of the two sides selected. Then at two ends of a 4-side-chain we leave out three sides more. Hence 4 sides of the 4-side-chain plus six more sides (6 from each end) – in total 10 sides are deducted. The third side must be selected from the remaining 10 sides. This is true for all the twenty 4-side-chains. Hence we get = 200 triplets.

2. Exactly three sides between one pair of selected sides

Similarly we first count 5-side-chains. There 20 such chains. From each chain we take the first and the fifth member for our triplet.

The third member of the triplet can be selected in exactly two ways:

a) The third side is exactly 3-sides away from one of the two selected sides. Then we are basically counting 9-side-chains. There are 20 such chains leading to 20 triplets.

b) The third side is strictly MORE than 3-sides away from both of the selected sides. Hence we leave out 4 sides from each end of the 5-chain. Hence the 3^rd side has to be selected from the remaining 7 sides (20- 5 -4 -4) leading to = 140 triplets.

3. Exactly four sides between one pair of selected sides

First a 6-chain in selected from first and sixth side becomes member of the triplet. Investigation for the third member leads to two cases again.

a) Both gaps are of 4-side length. Hence we count all the 11-side-chains. There are 20 such chains leading to 20 triplets.

b) The third side is strictly MORE than 4 -side away from both of the selected sides. Hence we leave out 5 sides from each end of the 6-chain. Hence the 3^rd side has to be selected from the remaining 4 sides (20- 6 -5 -5) leading to = 80 triplets.

4. Exactly five sides between one pair of selected sides

First a 7-chain in selected from which first and seventh side becomes member of the triplet. Investigation for the third member leads to two cases.

a) Both gaps are of 5-side length. Hence we count all the 13-side-chains. There are 20 such chains leading to 20 triplets.

b) The third side is strictly MORE than 5 -side away from both of the selected sides. Hence we leave out 6 sides from each end of the 7-chain. Hence the 3^rd side has to be selected from the remaining 1 sides (20- 7 -6 -6) leading to = 20 triplets.

Number of triplets= 20 + 200 + 20 + 140 + 20 + 80 + 20 + 20 = 520

ANSWER: 520

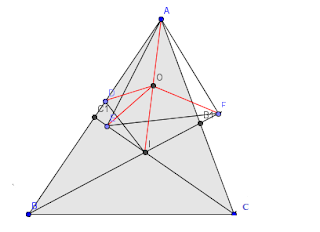
5. ABC is a triangle. \(BB_1\) and \(CC_1\) respectively are the bisectors of ∠B and ∠C with \(B_1\) on AC and \(C_1\) on AB. Let E anf F be the feet of the perpendiculars drawn from A onto \(BB_1\) and \(CC_1\) respectively. Suppose D is the point at which the incircle of ABC touches AB. Prove that AD = EF.

Solution:

Diagram:


Given: ABC be a triangle. \(BB_1\) and \(CC_1\) respectively are the bisectors of ∠B and ∠C with \(B_1\) on AC and \(C_1\) on AB. Let E anf F be the feet of the perpendiculars drawn from A onto \(BB_1\) and \(CC_1\) respectively. Suppose D is the point at which the incircle of ABC touches AB.

Required to Proof: AD = EF;

Construction: Let \(BB_1\) and \(CC_1\) intersect at I which is then the incentre of the triangle. ID is the perpendicular dropped on AB from I. Then ID is an inradius. EF and AI are joined. Let O be the midpoint of AI. OD, OA, OE and OF are joined.


Proof: 


∠ADI = ∠AEI (by given hypothesis both the right angles)
=> A, I, E, D concyclic (since angles subtended by the segment AI at D and E are equal)
=> Also AI is the diameter
Similarly A, I, E and F are concyclic.
But one and only one circle can pass through three non collinear points. Hence only one circle passes through A, I and E.
Hence all the five points A, I, E, D and F are on the same circle which has AI as the diameter.
Since O is the midpoint of AI and AI is the diameter; hence O is the center of the circle through A, D, E, I, F.

In triangle ΔOAD and ΔOEF
OD = OE (radii of the same circle)
OA = OF (radii of the same circle)
∠AOD = ∠EOF = 180° - A (proof of this claim follows)

Hence ΔOAD  ≅ ΔOEF => AD = EF (HENCE PROVED)


Lemma
R.T.P.: ∠AOD = ∠EOF = 180° - ∠A
Proof: ∠AOD = 2∠AID (angle at the circumference is half the angle at the center).

Also ∠AID = 90° - ∠A/2 (since I is the incenter, AI bisects ∠A; hence in ΔADI, ∠ADI = 90°, ∠DAI = ∠A/2; remaining ∠AID = 90° - ∠A/2)

Hence  ∠AOD = 2∠AID = 2(90° - ∠A/2) = 180° - ∠A -- (*)

Again in  ΔIBC, ∠IBC = ∠B/2; ∠ICB = ∠C/2; hence remaining ∠BIC = 180° - (∠B/2+ ∠C/2) = 90° + ∠A/2; Hence ∠EIF = 90° + ∠A/2 (vertically opposite angles);
Hence ∠EAF = 90°- ∠A/2 (Since EAFI is a cyclic quadrilateral, sum of opposite angles ∠EIF and ∠EAF is 180°)
Thus  ∠EOF = 2∠EAF = 180° - ∠A (Since angle at the center is twice the angle at the circumference) -- (**)

Hence from  (*) and (**) we conclude ∠AOD = ∠EOF = 180° - ∠A

Q.E.D. 

6. Find all pairs \((x,y) \in \mathbb{R} \) such that \(16^{x^2 + y} + 16^{x + y^2} \) =1

Solution: